
My Understanding of Collaborative and Problem-Solving Approaches to Learning Mathematics
I have almost two decades of Mathematics Textbook and Reference books writing and 3 decades of teaching experience in schools in Nepal. Bringing in the references from these experiences, this paper discusses my experiential understanding of collaborative and problem-solving approaches to learning mathematics. Also, it critically reflects on the learning and teaching practices that I have experienced during different levels of school and university. The first half of the paper is about my understanding of the concept. In the second half, I make a practitioner reflection on my experiences with collaborative (and problem-solving) approaches to learning mathematics.
Collaborative Approaches to Learning Mathematics
Growing up in schools where teaching and learning mathematics was largely lecture-based (Luitel, 2013), I started teaching mathematics in similar ways. As a teacher, I would enter the classroom to ‘teach’ students. I would ask them to ‘hear’ me, and ‘see’ what I would write on the board. Later, my higher study engagements at the university enabled me to see Mathematics teaching and learning as a collaborative and contextually relevant process (Shrestha, 2019). For example, I began to see mathematics not as a ‘pure’ and ‘foreign’ subject’ (See Luitel, 2013), but as something applicable and life-based. If so, I had to start teaching mathematics in a way that my teaching could enable students to learn in an authentic environment, and enable them to contextualize lessons in their everyday life.
Arriving at this stage, I have begun to understand that, unlike lecture-based teaching and learning, students work in groups as part of a collaborative teaching strategy. It is a strategy teachers employ when putting students in groups to improve learning (Swisher, 1990). I am in line with those researchers who support collaborative learning in ways that it benefits students in a variety of ways. It motivates the students to communicate with one another and use one another as resources. In this approach, teachers make sure there are plenty of chances for students to relate to each other and set up peer learning. These opportunities can be a tutoring session, peer instruction, or a time when both students contribute equally. For example, in math, pairing up a weak student with a talented student and an average student helps to boost learning achievement because learning with friends is easier than learning with a teacher (McPhee, 2002). As I have experienced, collaborative mathematics learners bring strengths to the table and learn from each other as they work. The use of group work in the classroom makes students get together for a simple, quick learning activity, such as playing a game or creating a chart.
In my experience, this concept of collaborative learning centers its learning activities on four key ideas: First, active learning is best for kids. Active engagement, such as moving about, drawing, creating, and performing, is a key component of collaborative learning. Second, in collaborative learning, instruction is student-centered rather than content-centered. This means, as a collaborative facilitator, I need to think about my pupils’ learning styles and the best ways to support them when I plan my lessons. Third, from a peer or group of peers, students can sometimes learn more quickly and effortlessly. Doing so also enhances teacher-led instruction by providing problem-solving opportunities (Swisher, 1990). And fourth, in problem-solving spaces, children have the opportunity to tackle issues jointly or with other group members when working with other pupils to complete tasks like a lengthy group project or a word problem in math. In this regard, I like collaborative teaching and learning in a way that students gain useful social interaction skills including compromise, teamwork, and the ability to listen to and understand others.
Collaborative Learning: Why Use It?
Also, I got an opportunity to study some research works on collaborative learning in Mathematics. According to research, students who collaborate acquire higher-order thinking skills, are better time managers and are more likely to be leaders (Capon & Kuhn, 2004). Students that work in groups appear to be more responsible and have higher self-esteem than those who don’t. Students become more understanding and sympathetic through collaborative learning. Tolerance is taught by interacting with students from other races, ethnicities, and backgrounds (McPhee, 2002). In this approach, the teacher prepares the students with scenarios where they need to spend their days communicating with friends, family, and coworkers. Peer tutoring and group projects make collaborative learning meaningful. Students take benefit much from these social opportunities for learning.
As a teacher, I have realized that in collaborative teaching and learning, even teachers experience the advantages of group learning every day. Often, I feel good about equipping students with skills they can use in their future careers, whether they are in offices, dorm rooms, or any other type of workplace. One condition, however, is that- as a collaborative teacher, I need to be aware of how critical it is to get along with others and support a team.
Problem-Solving Approaches to Learning Mathematics
As mentioned above, this paper is about my reflective understanding of collaborative learning and problem-solving approaches to teaching and learning mathematics. Now, in a couple of paragraphs below, I discuss my changing perspectives and newly emerging understandings of problem-solving approaches to teaching and learning mathematics. I understand the world is full of math issues of all kinds. Making an effort to solve problems is not always simple. We are all aware that some mathematical problems have clear-cut, obvious solutions. However, some issues call for greater mathematical knowledge and intellect to resolve.
As a teacher, often I have pictured the mental struggle a student goes through when they see a problem, they wish to address but are unsure of where to start. Without the assistance of some facilitators, it can be very difficult for students to be directed by inquisitive questions toward solving mathematical problems (Albanese & Mitchell, 1993). In my experience, the key to problem-solving is individual guidance that leads students through a critical thinking process.
It is important that in problem-solving approaches as well, the students work in pairs and/or small groups or collaborative learning (Capon & Kuhn, 2004). This is how I have changed my recent practices. For example, I bring collaborative learning and problem solving approaches together. In my recent practices, first, they read and reread the problem to understand what it is about, what it is asking for, and it is context. Thereafter, as a facilitator, I ask them to describe or put the problem in their own words. Also, I ask them to visualize what the problem looks like. It enables students to identify goals to solve the problem (Schwartz et al., 2001). Thereafter, I ask them to use appropriate resources, such as math and standard dictionaries, etc. to assist them in correct math problem comprehension. Passing through all these stages, students begin to recognize if there are any conditions and/or assumptions that need to be applied to the math problem to receive commonsense results.
Also, continuing with problem-solving approaches, sometimes, I let students develop their plans with additional strategies. A visual aid may help the students use logical reasoning to assist in eliminating possibilities. In my experience, organized, organized lists could be used to help them stay focused. They can substitute values by using a formula. In the process, the students actively guess and check their estimates and at times work backward to understand the process (McPhee, 2002). The practices have enhanced my confidence as a mathematics teacher in a way that effective guidance can teach the approach to solve math problems and make the process easier for students.
My Experiences with Collaborative and Problem Solving and Approaches to Learning Mathematics
In reference to the above-discussed theoretical and conceptual understanding on collaborative learning and problem-solving approaches to teaching mathematics, here follows an example of a class I prepared to teach. Here, the class runs from ‘I do’ to ‘we do’ to ‘you do together’ to ‘you do alone’ approach to teaching and learning. While designing the lesson plan, I have integrated both the collaborative and problem-solving approaches.
Objective
By the end of the lesson, students will be able to explain how to buy an object each valued under 100 rupees accoutring two different ways.
Model 1: ‘I’ ‘Do’
Let us consider the following question:
The ice cream as shown in the picture at the right costs Rs 86. In what different ways do you use the following currencies to buy the ice-creams?


Image source: (Simkhada, 2023)
My way of explaining (one way):
Dear students,
- This ice cream cone costs Rs 86. We need to make Rs 86 in two different ways.
- Here my problem is how can I make Rs. 86?
- Let’s find the solution of this problem:
(I continue to solve the problem myself without giving students space to exercise their mind.)
First way: Rs. 50 + Rs 20 + Rs 10 + Rs 5 + Re 1
Second way: Rs. 50 + Rs 25 + Rs 10 + Re 1
In this model, I explain only the process of making Rs 86. I focus the lesson on teacher-centered
(Lecture) model. The role of students is passive listening. Here my teaching strategy is ‘I Do’.
Model 2: “We Do”
In this model, I follow the guided instruction with scaffolding, vocabulary and feedback.
My way of teaching is as follows (Interactive):
- I start with the question; what different currencies are there and what are their values?
- I present the question in the class and try to find the solution by participating students.
- I probe the question; can I start with Rs 50 to make Rs 86? if not why?
- Let’s take Rs 50 first. Then can I take other 50 to make 86? if not why?
- Let’s take Rs 50 and Rs 20. Now, is it 86? If not, what other currencies do we need to add?
- I continue similar questions until the solution is found in many ways.
(I give students, space to exercise their minds.)
In this way, I participate with the students to find the solution. I ask students another alternative method of solving this question and support them in finding the solution.
First way: Rs. 50 + Rs 20 + Rs 10 + Rs 5 + Re 1
Second way: Rs. 50 + Rs 25 + Rs 10 + Re 1
In this method, I as a facilitator, and the students are active in finding the solution to the problem. Here, my role is to probe questions to the students, and the student’s role is to actively participate.
Model 3: Collaborative learning model “you do together”
In this model ‘you do together’ means we divide the students into small groups and learning in pairs is emphasized.
Here, I modified the previous questions as follows:
Question:
There are different items with different price tags. You have sample money to buy the items.

- I start with the division of groups and instruction for the activity.
- I keep an item with price tag and the sample money of Re 1, Rs 5, Rs 10, Rs 20, Rs 25 and Rs 50 on the table for each group.
- I ask the students price of the item showing them the price tag.
- I ask the students the value of each currency note.
- Students begin to discuss in pairs.
- I actively observe their discussion.
Group A
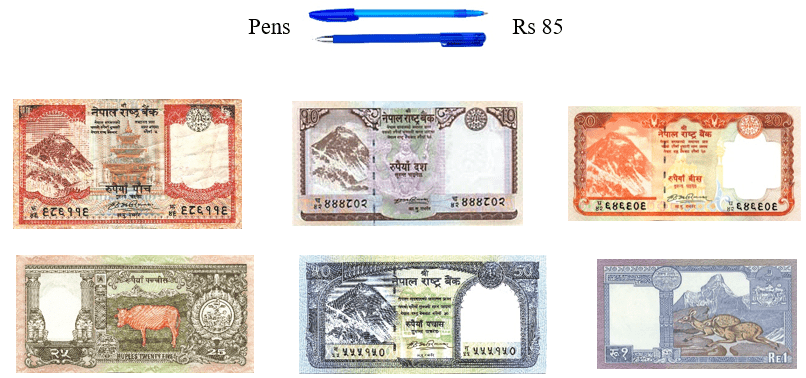
Image source: (Simkhada, 2023)
Group B
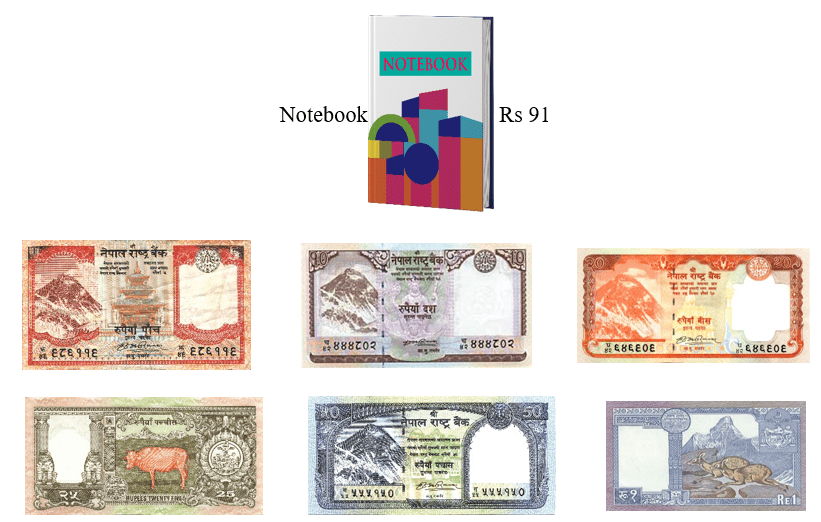
Image source: (Simkhada, 2023)
Group C

Image source: (Simkhada, 2023)
Group D
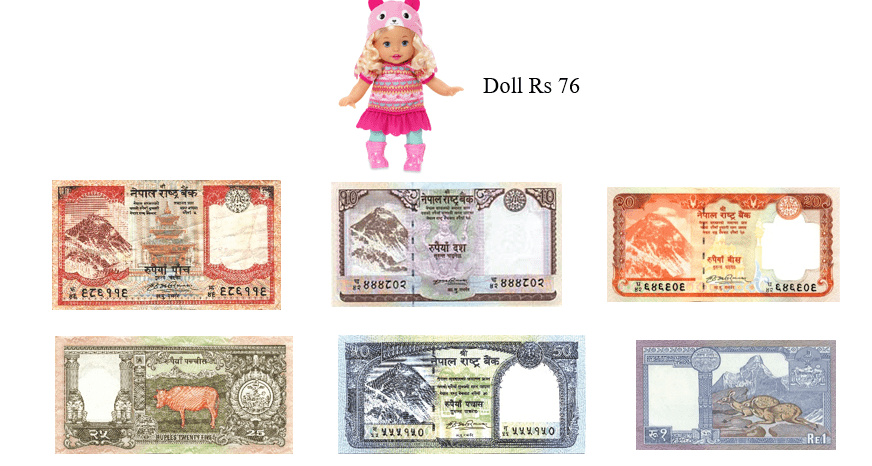
Image source: (Simkhada, 2023)
- Students discuss with each other how to make the exact money to buy the items in different ways.
- Students will fully engage to find the solution to the problem.
- My role will be to facilitate and support if help is required.
- Groupwise discussion of their ideas of making money in different ways makes learning more effective.
This method of learning is known as the collaborative learning model. It is also called the progressive learning model.
Model 4:”you do alone”
In this model, students solve the problem independently to meet the learning objectives.
- I prepare the different parallel question papers for each student and assign them individual work.
- In this model, the responsibility is shifted to the individual student.
Students can complete the objective independently without guidance from the teacher or each other. It is like the standardized test done in the examination practices in Nepal like BLE/SEE etc. But to reach at this stage the previous three stages or models have their own importance. Unfortunately, these stages are often missed in teaching-learning practices in Nepali schools.
My Concluding Insights from the Experience
From the above-discussed examples, I have developed an insight that a maths teacher can integrate both collaborative and problem-solving approaches in a single unit and enhance the strength of mathematics teaching and learning. In a collaborative learning group, members appreciate each other’s contributions, which makes working together fun. Because they share authority and have a great deal of respect for one another, they cooperate willingly and hold each other accountable. It entails more than just teamwork. It entails cooperating on a task or objective as a group in a structured activity that allows for participation from every group member. Cooperation abilities, problem-solving skills, and conflict resolution are all enhanced by collaborative learning. But, unlike the suggestions in some literature, I strongly believe that collaborative and problem-solving approaches to teaching and learning mathematics seek more active teachers as facilitators. Until and unless such teaching and learning become intentional and multi-dimensional (like ‘I do’, ‘we do’, ‘you do together’, and ‘you do alone’), there is always a risk that the approach becomes less effective.
References
Albanese, M. A., & Mitchell, S. (1993). Problem-based learning: A review of literature on its outcomes and implementation issue. Academic Medicine, 68, 52-81.
Capon, N., & Kuhn, D. (2004). What’s so good about problem-based learning? Cognition and Instruction, 22, 61–79.
Luitel, B.C. (2013). Mathematics as an im/pure knowledge system: Symbiosis (w)holism and synergy in mathematics education. International Journal of Science and Mathematics Education 10(6), 65-87. https://doi.org/10.1007/s10763-012-9366-8
McPhee, A. D. (2002). Problem-based learning in initial teacher education: Taking the agenda forward. Journal of Educational Enquiry, 3, 60– 78.
Schwartz, P., Mennin, S., & Webb, G. (Eds.). (2001). Problem-based learning: Case studies, experience and practice. London: Kogan Page.
Shrestha, I. M. (2019). Facilitating culturally de/contextualised mathematics education: An arts-based ethnodrama. In P. C. Taylor & B. C. Luitel (Eds.), Research as transformative learning for sustainable futures: Global voices and visions (pp. 225 – 238). Brill Sense.
Simkhada, D. R. (2023). Maths Links for grade 2, Kathmandu, Readmore Publishers and Distributors
Simkhada, D. R. (2023). Maths Links for grade 3, Kathmandu, Readmore Publishers and Distributors
Swisher, K. G. (1990). Cooperative learning and the education of American Indian/Alaska Native students: A review of the literature and suggestions for implementation. Journal of American Indian Education, 29, 36-43.
